Lens (optics) facts for kids
A lens is a clear (transparent) object (like glass, plastic or even a drop of water) that changes the way things look by bending the light that goes through it. They may make things appear larger, smaller, or upside-down. Some places lenses are used include spectacles (eyeglasses), contact lenses, cameras, projectors, microscopes, telescopes, magnifying glasses, etc. Each eye also has a lens.
They work by refraction, or the bending of light.
Lenses have three main shapes. Each different shape changes the image (making it bigger or smaller). The three types are:
- Planar: flat
History
The word "lens" comes from the Latin word for "lentil," because the earliest convex lenses in common use were shaped like the bean.
The oldest written record of lenses is in the ancient Greek play by Aristophanes called "Clouds", where lenses were used with sunlight to make fire.
Galileo Galilei is commonly believed to have used lenses to make the first known refracting telescope. However, he merely adapted and improved a design he learned from Dutch lensmakers such as Hans Lippershey. He was probably the first to use telescopes to make accurate astronomical observations, though.
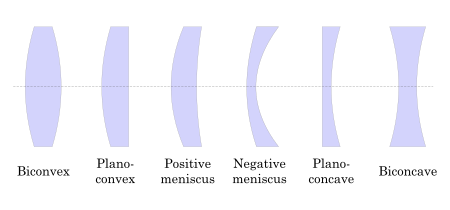
Types of simple lenses
Lenses are classified by the curvature of the two optical surfaces. A lens is biconvex (or double convex, or just convex) if both surfaces are convex. If both surfaces have the same radius of curvature, the lens is equiconvex. A lens with two concave surfaces is biconcave (or just concave). If one of the surfaces is flat, the lens is plano-convex or plano-concave depending on the curvature of the other surface. A lens with one convex and one concave side is convex-concave or meniscus. It is this type of lens that is most commonly used in corrective lenses, since its shape minimizes some aberrations.
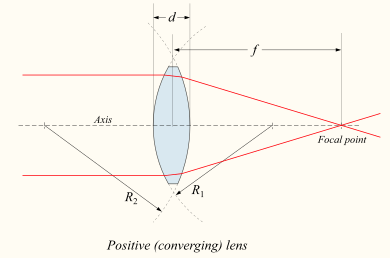
If the lens is biconvex or plano-convex, a collimated beam of light passing through the lens converges to a spot (a focus) behind the lens. In this case, the lens is called a positive or converging lens. For a thin lens in air, the distance from the lens to the spot is the focal length of the lens, which is commonly represented by f in diagrams and equations. An extended hemispherical lens is a special type of plano-convex lens, in which the lens's curved surface is a full hemisphere and the lens is much thicker than the radius of curvature.
Another extreme case of a thick convex lens is a ball lens, whose shape is completely round. When used in novelty photography it is often called a "lensball". A ball-shaped lens has the advantage of being omnidirectional, but for most optical glass types, its focal point lies close to the ball's surface . Because of the ball's curvature extremes compared to the lens size, optical aberration is much worse than thin lenses, with the notable exception of chromatic aberration.
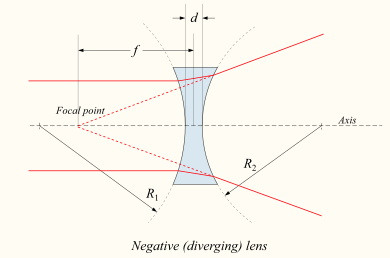
If the lens is biconcave or plano-concave, a collimated beam of light passing through the lens is diverged (spread); the lens is thus called a negative or diverging lens. The beam, after passing through the lens, appears to emanate from a particular point on the axis in front of the lens. For a thin lens in air, the distance from this point to the lens is the focal length, though it is negative with respect to the focal length of a converging lens.
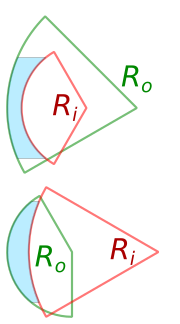
Convex-concave (meniscus) lenses can be either positive or negative, depending on the relative curvatures of the two surfaces. A negative meniscus lens has a steeper concave surface (with a shorter radius than the convex surface) and is thinner at the centre than at the periphery. Conversely, a positive meniscus lens has a steeper convex surface (with a shorter radius than the concave surface) and is thicker at the centre than at the periphery.
An ideal thin lens with two surfaces of equal curvature would have zero optical power, meaning that it would neither converge nor diverge light. All real lenses have a nonzero thickness, however, which makes a real lens with identical curved surfaces slightly positive. To obtain exactly zero optical power, a meniscus lens must have slightly unequal curvatures to account for the effect of the lens' thickness.
Related pages
Images for kids
-
Light being refracted by a spherical glass container full of water. Roger Bacon, 13th century
-
Close-up view of a flat Fresnel lens.
See also
 In Spanish: Lente para niños
In Spanish: Lente para niños