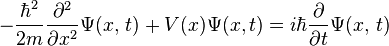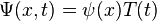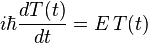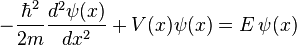Schrödinger equation facts for kids
The Schrödinger equation is a differential equation (a type of equation that involves an unknown function rather than an unknown number) that forms the basis of quantum mechanics, one of the most accurate theory of how subatomic particles behave. It is a mathematical equation that was thought of by Erwin Schrödinger in 1925. It defines a wave function of a particle or system (group of particles) which has a certain value at every point in space for every given time. These values have no physical meaning (in fact, they are mathematically complex), yet the wave function contains all information that can be known about a particle or system. This information can be found by mathematically manipulating the wave function to return real values relating to physical properties such as position, momentum, energy, etc. The wave function can be thought of as a picture of how this particle or system acts with time and describes it as fully as possible.
The wave function can be in a number of different states at once, and so a particle may have many different positions, energies, velocities or other physical property at the same time (i.e. "be in two places at once"). However, when one of these properties is measured it has only one specific value (which cannot be definitely predicted), and the wave function is therefore in just one specific state. This is called wave function collapse and seems to be caused by the act of observation or measurement. The exact cause and interpretation of wave function collapse is still widely debated in the scientific community.
For one particle that only moves in one direction in space, the Schrödinger equation looks like:
where 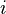 is the square root of -1,
is the square root of -1, 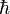 is the reduced Planck's constant,
is the reduced Planck's constant,  is time,
is time, 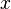 is a position,
is a position, 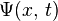 is the wave function, and
is the wave function, and 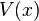 is the potential energy, an as yet not chosen function of position. The left hand side is equivalent to the Hamiltonian energy operator acting on
is the potential energy, an as yet not chosen function of position. The left hand side is equivalent to the Hamiltonian energy operator acting on  .
.
Time independent Version
Assuming that the wave function, 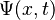 , is separable, i.e. assuming the function of two variables can be written as the product of two different functions of a single variable:
, is separable, i.e. assuming the function of two variables can be written as the product of two different functions of a single variable:
then, using standard mathematical techniques of Partial differential equations, it can be shown that the wave equation can be rewritten as two distinct differential equations
where the first equation is solely dependent on time  , and the second equation depends only on position
, and the second equation depends only on position  , and where
, and where  is just a number. The first equation can be solved immediately to give
is just a number. The first equation can be solved immediately to give
where  is Euler's number. Solutions of the second equation depend on the potential energy function,
is Euler's number. Solutions of the second equation depend on the potential energy function, 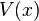 , and so cannot be solved until this function is given. It can be shown using quantum mechanics that the number
, and so cannot be solved until this function is given. It can be shown using quantum mechanics that the number  is actually the energy of the system, so these separable wave functions describe systems of constant energy. Since energy is constant in many important physical systems (for example: an electron in an atom), the second equation of the set of separated differential equations presented above is often used. This equation is known as the Time independent Schrödinger Equation, as it does not involve
is actually the energy of the system, so these separable wave functions describe systems of constant energy. Since energy is constant in many important physical systems (for example: an electron in an atom), the second equation of the set of separated differential equations presented above is often used. This equation is known as the Time independent Schrödinger Equation, as it does not involve  .
.
Images for kids
-
Complex plot of a wave function that satisfies the nonrelativistic Schrödinger equation with V = 0. In other words, this corresponds to a particle traveling freely through empty space.
-
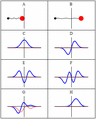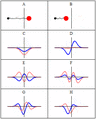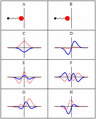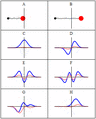
Each of these three rows is a wave function which satisfies the time-dependent Schrödinger equation for a harmonic oscillator. Left: The real part (blue) and imaginary part (red) of the wave function. Right: The probability distribution of finding the particle with this wave function at a given position. The top two rows are examples of stationary states, which correspond to standing waves. The bottom row is an example of a state which is not a stationary state. The right column illustrates why stationary states are called "stationary".
-
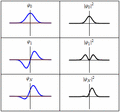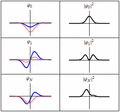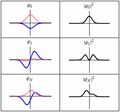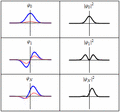
A harmonic oscillator in classical mechanics (A–B) and quantum mechanics (C–H). In (A–B), a ball, attached to a spring, oscillates back and forth. (C–H) are six solutions to the Schrödinger Equation for this situation. The horizontal axis is position, the vertical axis is the real part (blue) or imaginary part (red) of the wave function. Stationary states, or energy eigenstates, which are solutions to the time-independent Schrödinger equation, are shown in C, D, E, F, but not G or H.
See also
 In Spanish: Ecuación de Schrödinger para niños
In Spanish: Ecuación de Schrödinger para niños