Parabola facts for kids
The parabola (from the Greek παραβολή) is a type of curve. Menaechmus (380–320 BC) discovered the parabola, and Apollonius of Perga (262 BC–c190 BC) first named it.
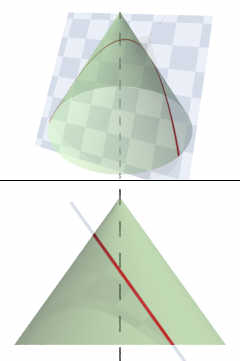
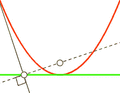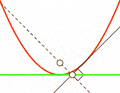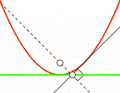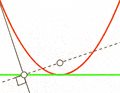
A parabola is a conic section. If a cone is dissected by a plane which is parallel to one of the surfaces of the cone, the result is a parabola. The point where the parabola reaches its maximum or minimum is called the "vertex." At this point the curvature of the parabola is greatest.
Each parabola has a focal point. Any ray that enters the parabola and is parallel to the axis of symmetry will pass through this point after being reflected by the curve. Because of this fact, parabolas are important in devices such as satellite dishes, or magnifying mirrors. Parabolas are often used to approximate curves that are more difficult to model by themselves.
Every parabola uses the equation 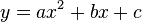 , where
, where  ,
, 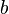 and
and  are constants, and
are constants, and  is not equal to
is not equal to 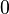 .
.
Images for kids
-
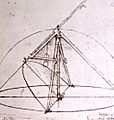
Parabolic compass designed by Leonardo da Vinci
-
A bouncing ball captured with a stroboscopic flash at 25 images per second. The ball becomes significantly non-spherical after each bounce, especially after the first. That, along with spin and air resistance, causes the curve swept out to deviate slightly from the expected perfect parabola.
-
The supporting cables of suspension bridges follow a curve that is intermediate between a parabola and a catenary.
-
The Rainbow Bridge across the Niagara River, connecting Canada (left) to the United States (right). The parabolic arch is in compression and carries the weight of the road.
-
Parabolic shape formed by a liquid surface under rotation. Two liquids of different densities completely fill a narrow space between two sheets of transparent plastic. The gap between the sheets is closed at the bottom, sides and top. The whole assembly is rotating around a vertical axis passing through the centre. (See Rotating furnace)
-
Array of parabolic troughs to collect solar energy
-
Edison's searchlight, mounted on a cart. The light had a parabolic reflector.
-
Physicist Stephen Hawking in an aircraft flying a parabolic trajectory to simulate zero gravity
See also
 In Spanish: Parábola (matemática) para niños
In Spanish: Parábola (matemática) para niños