Image: CalabiYau5

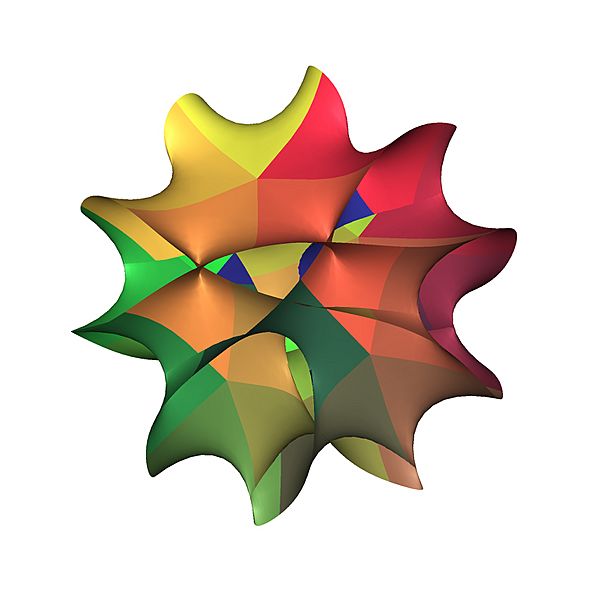
Description: This image shows a local 2D cross-section of the real 6D manifold known in string theory as the Calabi-Yau quintic. This is an Einstein manifold and a popular candidate for the wrapped-up 6 hidden dimensions of 10-dimensional string theory at the scale of the Planck length. The 5 rings that form the outer boundaries shrink to points at infinity, so that a proper global embedding would be seen to have genus 6 (6 handles on a sphere, Euler characteristic -10). The underlying real 6D manifold (3D complex) has Euler characteristic -200, is embedded in CP4, and is described by this homogeneous equation in five complex variables: z05 + z15 + z25 + z35 + z45 = 0 The displayed surface is computed by assuming that some pair of complex inhomogenous variables, say z3/z0 and z4/z0, are constant (thus defining a 2-manifold slice of the 6-manifold), renormalizing the resulting inhomogeneous equations, and plotting the local Euclidean space solutions to the inhomogenous complex equation z15 + z25 = 1 This surface can be described as a family of 5x5 phase transformations on a fundamental domain, 1/25th of the surface, shown (slightly hidden) in blue. Each of the first set of phases mixes in a brighter red color to its patch, and the second set mixes in green. Thus the color alone shows the geometric parentage of each of the 25 patches. The resulting surface, which is embedded in 4D, is projected to 3D according to one's taste to produce the final rendering. Further details are given in Andrew J. Hanson, "A construction for computer visualization of certain complex curves," Notices of the Amer. Math. Soc. 41 (9): 1156-1163, (November/December 1994).
Title: CalabiYau5
Credit: OTRS Ticket#2014010910010981
Author: Andrew J. Hanson
Permission:   This work is free and may be used by anyone for any purpose. If you wish to use this content, you do not need to request permission as long as you follow any licensing requirements mentioned on this page. Wikimedia has received an e-mail confirming that the copyright holder has approved publication under the terms mentioned on this page. This correspondence has been reviewed by an OTRS member and stored in our permission archive. The correspondence is available to trusted volunteers as ticket #2014010910010981. If you have questions about the archived correspondence, please use the OTRS noticeboard. Ticket link: https://ticket.wikimedia.org/otrs/index.pl?Action=AgentTicketZoom&TicketNumber=2014010910010981
Usage Terms: Creative Commons Attribution-Share Alike 3.0
License: CC BY-SA 3.0
License Link: http://creativecommons.org/licenses/by-sa/3.0
Attribution Required?: Yes