Conway knot facts for kids
Quick facts for kids Conway knot |
|
|---|---|
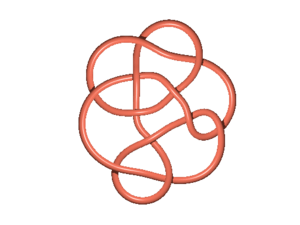 |
|
| Crossing no. | 11 |
| Genus | 3 |
| Conway notation | .-(3,2).2 |
| Thistlethwaite | 11n34 |
| Other | |
| prime, prime | |
In mathematics, in particular in knot theory, the Conway knot (or Conway's knot) is a particular knot with 11 crossings, named after John Horton Conway.
It is related by mutation to the Kinoshita–Terasaka knot, with which it shares the same Jones polynomial. Both knots also have the curious property of having the same Alexander polynomial and Conway polynomial as the unknot.
The issue of the sliceness of the Conway knot was resolved in 2020 by Lisa Piccirillo, 50 years after John Horton Conway first proposed the knot. Her proof made use of Rasmussen's s-invariant, and showed that the knot is not a smoothly slice knot, though it is topologically slice (the Kinoshita–Terasaka knot is both).
See also
 In Spanish: Nudo Conway para niños
In Spanish: Nudo Conway para niños

All content from Kiddle encyclopedia articles (including the article images and facts) can be freely used under Attribution-ShareAlike license, unless stated otherwise. Cite this article:
Conway knot Facts for Kids. Kiddle Encyclopedia.


